The selective serotonin reuptake inhibitors (SSRIs) significantly advanced the treatment of mood disorders, anxiety disorders, and other psychiatric disorders through improved tolerability, more efficient dosing, and equal efficacy when compared with the tricyclic antidepressants (
1). Primary care physicians now treat uncomplicated forms of common psychiatric disorders without psychiatric referral.
With growing emphasis on cost containment and because SSRI acquisition costs represent substantial expenditures for hospitals and health plans, attention to the cost-effectiveness of antidepressants has taken on increased importance. However, relatively few data are available to help make the best decisions on which agents to select for a patient population formulary.
In a previous outcomes study on the effectiveness of antidepressants, the SSRIs were equivalent as an optimal drug of first choice (
2). Regardless of the initial choice of SSRI and in the absence of specific case considerations, between 15 and 25 percent of the patients (22 percent overall) in that study who were started on an SSRI switched to another antidepressant in the course of their treatment. There were no overall differences in rates of changes of medications or outcomes between drugs within the SSRI class. These findings extend other studies that found no difference in switch rates when the SSRIs paroxetine, fluoxetine, and sertraline were compared with other antidepressants (
3,
4).
This report describes a method for modeling cost data for antidepressants from clinical outcome data, given equal overall efficacy and effectiveness among the antidepressant agents and lacking patient markers specifying selection of a particular antidepressant agent. The method is demonstrated here using data from a large clinical outcomes study on the three most frequently used SSRIs—paroxetine, fluoxetine, and sertraline (
2). When the consequences of the alternatives being compared are equivalent, cost minimization is the special form of cost-effectiveness analysis that identifies the least expensive alternative (
5).
Methods
Subjects and setting
The sample consisted of 2,779 patients served over the 22-month period from March 1995 to January 1997 at the general clinic at the University of New Mexico Mental Health Center in Albuquerque. The facility is an outpatient psychiatry teaching clinic that provides comprehensive diagnostic assessment with psychosocial and medication management to a primarily public-sector population. The clinic treats up to 30 new patients and 120 follow-up patients every week and has an active caseload of about 500 patients. More than 1,500 patients are treated each year.
The clinic database includes information on patients' primary
DSM-IV axis I, II, and III diagnoses, treatment duration, visits, medications, dosages, admission and discharge score on the Clinical Global Impressions severity-of-illness scale, disposition, and admission and discharge scores on the Symptom Checklist-90. Further details about recruitment, diagnoses, procedures, and outcomes are provided elsewhere (
2).
Pharmacoeconomic analysis
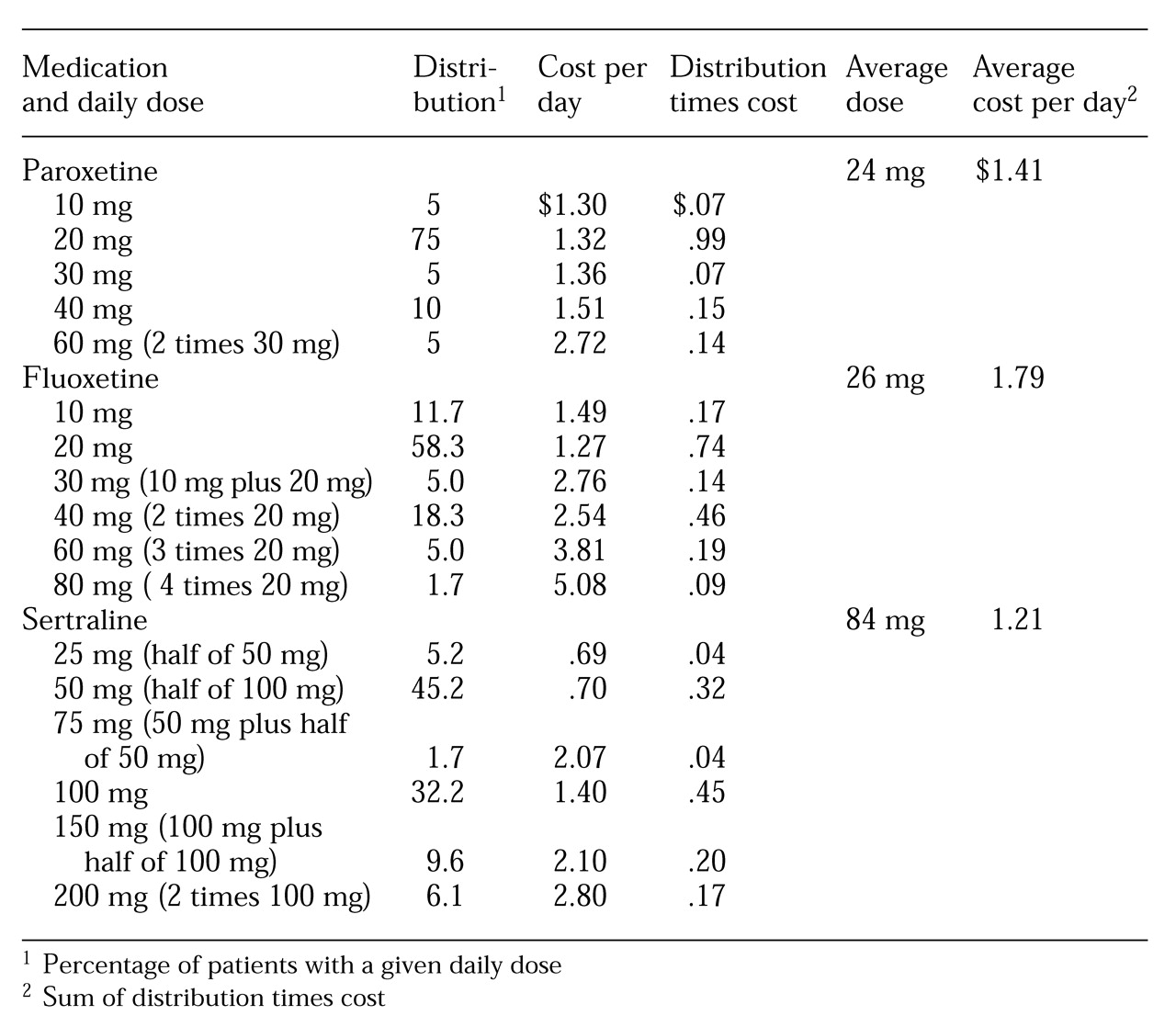
Individual patients take specific rather than average doses. Actual average drug costs per day must be calculated by determining costs for the percentage of patients receiving each daily dose and then summing the costs in a procedure known as dose stratification. The percentage of patients receiving each dose—the distribution of the doses—is multiplied by the cost of that medication dose to produce the cost per day. The sum of the multiplication products determines the true average cost per day of the medication.
For example, for medication A, 70 percent of patients take 20 mg and 30 percent take 30 mg per day. A 20-mg tablet costs $1.45, and 30 mg tablet costs $2.10. The actual average cost per day is determined by multiplying .70 by $1.45 to obtain $1.02, multiplying .30 by $2.10 to yield $.63, and then adding the products together to get $1.65.
This method contrasts with the incorrect but commonly used method that uses the cost of tablet sizes relative to average doses and results in an average cost per milligram (cost times average dose divided by tablet size). In the previous example, if the average dose of medication A is 23 mg, this method results in a reported average cost per day of 23 mg times $1.45 divided by 20 mg or $1.67.
In the method we describe, actual acquisition costs are used for determining comparative costs. In the examples used in this report, the acquisition costs were obtained from the pharmacy at the University of New Mexico Health Sciences Center. The center serves a high percentage of indigent patients and thus qualifies for disproportionate share pricing, an established discount below average wholesale price. In addition, the pharmacy uses half of 100 mg tablets for 50 mg sertraline doses.
Results
Antidepressant medications were prescribed for 77 percent of the 2,779 patients, or 2,140 patients. Eighty-one percent of the patients who received an antidepressant—a total of 1,733 patients—received an SSRI; 9 percent, or 193 patients received a novel antidepressant; and 10 percent, or 214 patients, received a tricyclic antidepressant. Of the patients who were prescribed SSRIs, 28 percent, or 485 patients, received fluoxetine; 18 percent, or 317 patients, received paroxetine; and 54 percent, or 931 patients, received sertraline. Mean doses with 95 percent confidence intervals were 26 mg (CI=23 to 33 mg) for fluoxetine, 24 mg (CI=21 to 26 mg) for paroxetine, and 84 mg (CI=76 to 92 mg) for sertraline. Seventy percent of the patients who received fluoxetine and 80 percent of the patients who received paroxetine completed treatment with a final dose of 20 mg or less. Among patients who received sertraline, 84 percent had a final dose of 100 mg or less, and 51 percent had a final dose of 50 mg or less.
Table 1 shows the actual average drug cost per day for each of the three SSRIs determined by calculating the costs for the percentage of patients on each daily dose (sum of distribution times cost divided by day). The average costs per day using this method are $1.79 for fluoxetine, $1.41 for paroxetine, and $1.21 for sertraline.
These results contrast with those that would be obtained from the common but incorrect approach using cost per milligram of the average dose (cost times average dose divided by tablet size). That method would report average costs per day of $1.68 for fluoxetine using 20 mg tablets, $1.58 for paroxetine using 20 mg tablets, $1.17 for sertraline using 100 mg tablets, and $2.32 for sertraline using 50 mg tablets.
Discussion and conclusions
Several limitations of the generalizability of these results should be mentioned. The naturalistic method for assessing medication effectiveness does not substitute for a randomized controlled efficacy study in which patients are systematically assessed and assigned to study groups in terms of diagnosis, outcome measures, SSRI used, and dose titration. There was no difference in the outcome variables, with the exception that patients who switched medication were in treatment 40 percent longer than those who did not switch.
Levels of sertraline use may have been higher to the extent that patients were referred or returned to the primary care setting in which sertraline is the primary SSRI in the institutional formulary. An ascertainment bias affecting dosages must be considered because this clinic is an academic tertiary care center serving a population with lower socioeconomic status and with potentially greater illness severity and comorbidity. Given these parameters, the SSRI dosage means and use of halved 100 mg tablets of sertraline are consistent with reports from other settings (
6,
7). If 50 mg tablets of sertraline were used instead of halved 100 mg tablets, the average cost of sertraline would be $.35 higher.
Costs and dosage distributions reported here were used only to demonstrate a correct pharmacoeconomic cost-minimization analysis. Other settings should use their own medication acquisition costs and dose distributions to determine comparisons. We emphasize that no particular SSRI is being promoted.
The stratification methodology correctly determines average drug cost per day by calculating the percentage of patients on each daily dose. In the study setting described here, this analysis demonstrated that using halved 100 mg tablets of sertraline for 50 mg doses is less expensive ($1.21 per day) than using paroxetine ($1.41) or fluoxetine ($1.79). The average sertraline cost is determined correctly—costs for both 50 mg and 100 mg tablets are taken into account rather than considering different costs for each size of tablets in determining the cost per mg relative to average dose in the sample. Drug costs based on the cost per milligram of average doses are misleading because they fail to account for different pill costs as applied to patient dose distribution. In the sample reported here, this method would overestimate the actual costs of using 50 mg tablets of paroxetine and sertraline and underestimate the cost of using 100 mg of fluoxetine and sertraline.
Due to competitive market forces, practitioners and others who are responsible for drug acquisition are confronted with an array of claims of effectiveness when selecting an SSRI for patients. In the economic arena, those who attempt to illustrate the highly contentious issue of a medication's cost-efficiency frequently embrace unproven methodologies or use methods that cost out direct expenses, such as those for drugs, clinical visits, and laboratory tests, along with indirect expenses such as loss of life, earnings, and productivity. Often what appear to be unbiased analyses serve a less apparent agenda. Due to the complexity of such methods, they are difficult to assess critically.
We suggest that until superior clinical efficacy and effectiveness can be demonstrated conclusively for a particular SSRI or other antidepressant, acquisition costs, if analyzed correctly, can help guide the choice of agent for a population when indicators for use of a specific agent are absent.
Acknowledgment
The authors thank Michael P. Dutro, Pharm.D., for technical assistance and advice during the study.